步骤:
1. \dot x =A*x + B*u is a state space model, with A and B are known. Now we want to locate the poles p at the desired location, so need the help with designing a controller: u= - k*x。How to choose the gain k?
Answer: Substituting u= - k*x, then \dot = (A-B*K)x, use place function in Matlab to input (A,B,p), obtaining k from the output.
About the introduction of place function, I attach a paragraph from Wikipedia here:
Description
Given the single- or multi-input system
˙x=Ax+Bu
and a vector
pof desired self-conjugate closed-loop pole locations,placecomputes a gain matrixKsuch that the state feedback u = –Kx places the closed-loop poles at the locationsp. In other words, the eigenvalues of A – BK match the entries ofp(up to the ordering).
K = place(A,B,p)places the desired closed-loop polespby computing a state-feedback gain matrixK. All the inputs of the plant are assumed to be control inputs. The length ofpmust match the row size ofA.placeworks for multi-input systems and is based on the algorithm from [1]. This algorithm uses the extra degrees of freedom to find a solution that minimizes the sensitivity of the closed-loop poles to perturbations in A or B.
[K,prec,message] = place(A,B,p)returnsprec, an estimate of how closely the eigenvalues of A – BK match the specified locationsp(precmeasures the number of accurate decimal digits in the actual closed-loop poles). If some nonzero closed-loop pole is more than 10% off from the desired location,messagecontains a warning message.You can also use
placefor estimator gain selection by transposing theAmatrix and substitutingC'forB.l = place(A',C',p).'
Examples
Pole Placement Design
Consider a state-space system
(a,b,c,d)with two inputs, three outputs, and three states. You can compute the feedback gain matrix needed to place the closed-loop poles atp = [-1 -1.23 -5.0]byp = [-1 -1.23 -5.0]; K = place(a,b,p)
2. How to design the desired poles?
Answer: Choose a suitable value of \omega (bandwith) and \zeta (damping) of the controlling system:
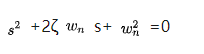
Then use the roots function to input the \omega and \zeta, obtaining the roots (the poles) as the output.
About the introduction of roots function, I attach a paragraph from Wikipedia here:
The
rootsfunction calculates the roots of a single-variable polynomial represented by a vector of coefficients.For example, create a vector to represent the polynomial x2−x−6, then calculate the roots.
p = [1 -1 -6]; r = roots(p)
r =
3
-2
Then with poles and system matrix (A, B), we can calculate the gain k.