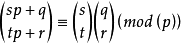Lucas定理在ACM中的应用

首先，Lucas（卢卡斯）定理是什么？有什么用？

Lucas定理是用来求 C(n,m) mod p，p为素数的值。（注意：p一定是素数）

有人会想，C(n,m)不能用C(n, m) = C(n - 1，m) + C(n - 1, m - 1)的公式来递推吗？

（提示：C(n, m) mod p = n!/(m!(n - m)!) mod p ）

可以是可以。但当n，m，p都很大时，你递推所用的时间就会很爆炸了。所以，这就需要用到Lucas定理来解决了。

因此，Lucas定理用来解决大组合数求模是很有用的。

注意：Lucas定理最大的数据处理能力是p在10^5左右，不能再大了。再大的话，我就不知道了。。。智障啊

表达式：C(n,m)%p=C(n/p,m/p)*C(n%p,m%p)%p。（可以递归）

递归方程：(C(n%p, m%p)*Lucas(n/p, m/p))%p。（递归出口为m==0，return 1）

-----------------------------------------------------------------------

Lucas定理定义：：

这里我们令n=sp+q , m=tp+r .（q ，r ≤p）

那么：

（在编程时你只要继续对

调用Lucas定理即可。）

（代码可以递归的去完成这个过程，其中递归终点为 t = 0 ）

（时间复杂度 O（logp(n)*p) ：）

-------------------------------------------------------------------------------------------

Lucas定理证明：

首先你需要这个算式：

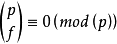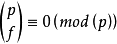，其中f > 0&& f < p。

然后(1 + x) nΞ(1 + x) sp+q Ξ( (1 + x)p)s· (1 + x) q Ξ(1 + xp) s· (1 + x) q(mod p) 。

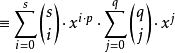
所以得(1 + x) sp+q

我们求右边的

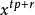的系数为：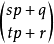

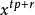
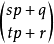
求左边的

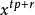为：

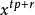
通过观察你会发现当且仅当i = t , j = r ,能够得到

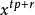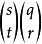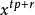的系数，及

。

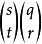所以，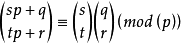

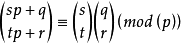。得证。

--------------------------------------------------------------------------------------------------

我再次将它公式化一下。

For non-negative integers m and n and a prime p, the following congruence relation holds:

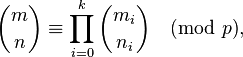
where

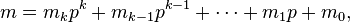
and

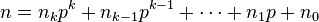
are the base p expansions of m and n respectively.

已知C(n, m) mod p = n!/(m!(n - m)!) mod p。显然是除法取模，这里又要用到m!(n-m)!的逆元。

求逆元（此处不详细说明了(ˇˍˇ) ）。。。

根据费马小定理：

已知(a, p) = 1，则 a^p-1 ≡ 1 (mod p), 所以 a*a^p-2 ≡ 1 (mod p)。

也就是 (m!(n-m)!)的逆元为 (m!(n-m)!)^{p-2 。}

按照上面的思路就可以写出Lucas定理的代码了：

#include <iostream>
using namespace std;
typedef long long ll;
ll N,M,fac[10010];
ll p=10007;

ll fast_pow(ll a,ll k,ll mod){
    ll ans=1;
    while(k){
        if(k&1) ans=(ans*a)%mod;
        a=(a*a)%mod;
        k>>=1;
    }
    return ans;
}

ll C(ll n,ll r){
    if(n<r)
        return 0;
    return fac[n]*fast_pow(fac[r]*fac[n-r],p-2,p)%p;
}

ll lucas(ll n,ll m){
    if(m==0)
        return 1;
    return (C(n%p,m%p)*lucas(n/p,m/p))%p;
}

int main(){
    fac[0]=1;
    for(int i=1;i<p;i++)
        fac[i]=fac[i-1]*i%p;
    while(cin>>N>>M)
        cout<<lucas(N,M)<<endl;
    return 0;
}

Lucas定理在ACM中的应用

猜你喜欢